
No such general formulas exist for higher degrees. So in conclusion, there are only general formulae for 1st, 2nd, 3rd, and 4th degree polynomials. It's that we will never find such formulae because they simply don't exist. So it's not that we haven't yet found a formula for a degree 5 or higher polynomial. The Abel-Ruffini Theorem establishes that no general formula exists for polynomials of degree 5 or higher. In fact, the highest degree polynomial that we can find a general formula for is 4 (the quartic).
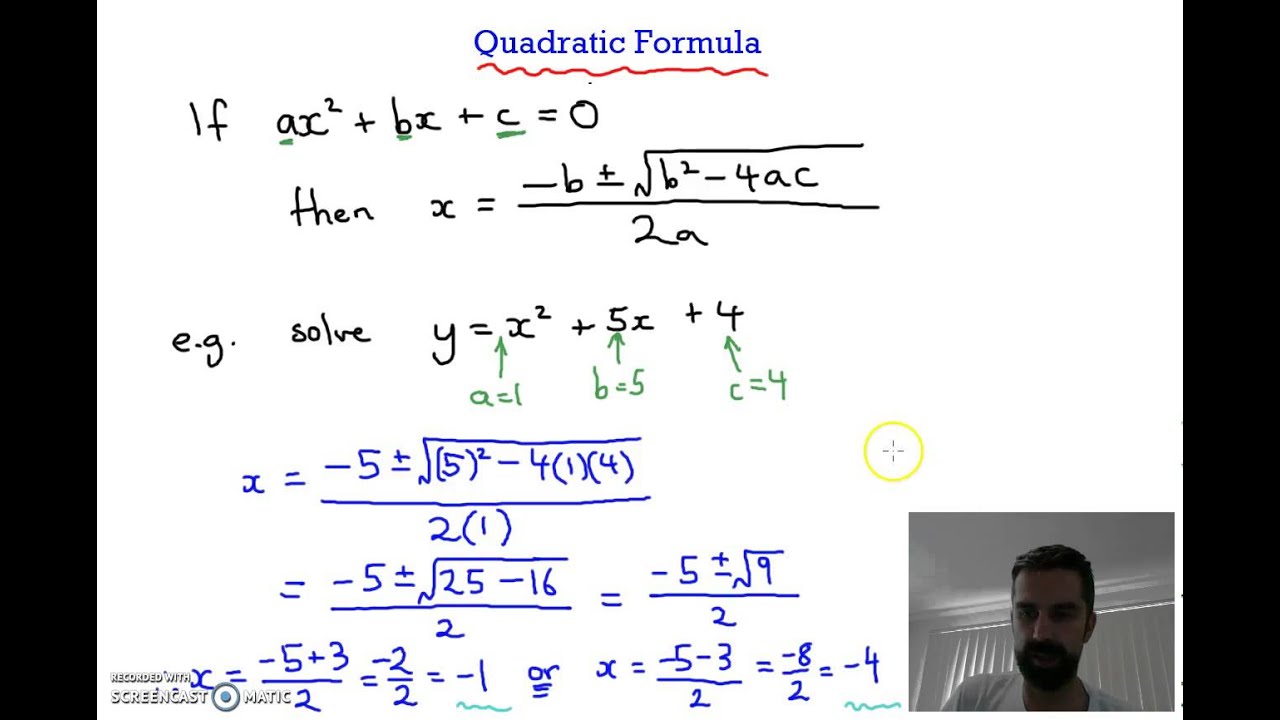
#Quadratic polynomial full#
Both of these formulas are significantly more complicated and difficult to derive than the 2nd degree quadratic formula! Here is a picture of the full quartic formula:īe sure to scroll down and to the right to see the full formula! It's huge! In practice, there are other more efficient methods that we can employ to solve cubics and quartics that are simpler than plugging in the coefficients into the general formulae. These are the cubic and quartic formulas. There are general formulas for 3rd degree and 4th degree polynomials as well. Similar to how a second degree polynomial is called a quadratic polynomial. A third degree polynomial is called a cubic polynomial. A trinomial is a polynomial with 3 terms. Factoring polynomials by taking common factors. 2 − 10 2 \frac 2 2 + 1 0 start fraction, 2, plus, square root of, 10, end square root, divided by, 2, end fractionįirst note, a "trinomial" is not necessarily a third degree polynomial. If asked for the exact answer (as usually happens) and the square roots can’t be easily simplified, keep the square roots in the answer, e.g. If you use a calculator, the answer might be rounded to a certain number of decimal places. Quadratic polynomials have the following properties, regardless of the form: It is a unicritical polynomial, i.e.Keep the + / − +/- + / − plus, slash, minus and always be on the look out for TWO solutions.Watch your negatives: b 2 b^2 b 2 b, squared can’t be negative, so if b b b b starts as negative, make sure it changes to a positive since the square of a negative or a positive is a positive.The solutions to this equation are called the roots. A univariate quadratic polynomial has the form f(x)a2x2+a1x+a0. In elementary algebra, such polynomials often arise in the form of a quadratic equation ax2 + bx + c 0. Make sure you take the square root of the whole ( b 2 − 4 a c ) (b^2 - 4ac) ( b 2 − 4 a c ) left parenthesis, b, squared, minus, 4, a, c, right parenthesis, and that 2 a 2a 2 a 2, a is the denominator of everything above it A quadratic polynomial is a polynomial of degree 2. The graph of a quadratic polynomial in a single variable is given by a parabola.If the quadratic polynomial is denoted as ax 2 + bx + c, then the equation of the parabola is y ax 2 + bx + c.Be careful that the equation is arranged in the right form: a x 2 + b x + c = 0 ax^2 + bx + c = 0 a x 2 + b x + c = 0 a, x, squared, plus, b, x, plus, c, equals, 0 or it won’t work! The discriminant of a quadratic polynomial, denoted ( Delta, ) is a function of the coefficients of the polynomial, which provides information about the properties of the roots of the polynomial.

Well also learn to manipulate more general polynomial expressions. For example,Ĥ x 2 + 2 x y − 3 y 2 īy a suitable choice of an orthogonal matrix S, and the diagonal entries of B are uniquely determined – this is Jacobi's theorem. Learn to factor expressions that have powers of 2 in them and solve quadratic equations. In mathematics, a quadratic form is a polynomial with terms all of degree two (" form" is another name for a homogeneous polynomial). For the usage in statistics, see Quadratic form (statistics). Step 2 Move the number term to the right side of the equation: P 2 460P -42000.


 0 kommentar(er)
0 kommentar(er)
